What is leading a group discussion in mathematics?
In a group discussion, the teacher and all of the students work on specific content together, using one another’s ideas as resources. The purposes of a discussion are to build collective knowledge and capability in relation to specific instructional goals and to allow students to practice listening, speaking, and interpreting. The teacher and a wide range of students contribute orally, listen actively, and respond to and learn from others’ contributions.
In a group discussion about mathematics, the teacher supports students to individually and collectively engage in sense-making about rich mathematical content. A mathematics discussion can provide opportunities for students to learn, practice, and refine habits of mind and discourse relevant to the field of mathematics.
How can leading a group discussion advance justice?
Teaching that advances justice requires that teachers attend to their students’ development, both as intellectual beings and as citizens. The free and fair exchange of ideas is a bedrock of a healthy democracy. Being an engaged world citizen requires the ability to share, justify and defend one’s ideas and – even more important – to listen attentively and thoughtfully to the ideas and perspectives of diverse others. Classrooms are an opportunity to practice the skills of reasoned argument, debate, and collective knowledge building toward common goals. Teachers can frame group discussions as opportunities for young people to make sense of something difficult together, and to support one another to both speak and listen in ways that advance the classroom community and common good.
By explicitly teaching disciplinary discourse norms and engaging students in discussions about mathematics, teachers can provide opportunities for students to have their voices heard, respected, affirmed, challenged and refined. When teachers do this deliberately, attending to students who have lower status or are likely to be marginalized, teachers can intervene to disrupt inequitable patterns of student participation. Teachers do this by intentionally positioning particular students as capable and competent publicly in front of their peers, calling attention to their strengths and contributions as assets in collaborative work (see Featherstone et al., 2011). This is especially critical in mathematics, a content domain where what counts as competence is often narrowly defined.
When this practice is routinely done well, students have repeated opportunities to formulate, revise, and refine mathematical arguments and as a result to come to see themselves as people who are capable of reasoning and making sense of mathematics using what they know to build new understandings. In addition, when all students are treated respectfully, when they are truly listened to, when their ideas are valued and understood as resources in instruction, and when these norms extend to how students treat one another, then a teacher’s work to lead a group discussion can serve as an opportunity to engender what Jo Boaler (2008) has termed relational equity. Like Boaler, we believe that when students experience equitable relations in mathematics classrooms, “the respect they learn to form for each other will impact the opportunities they extend to others in their lives in and beyond school” (p. 167).
How do we decompose the practice into learnable parts?
The practice of leading a group discussion is broken down into learnable and teachable components, as represented in the decomposition chart below. It is broken into two main areas of work: discussion enabling work and discussion leading work. Each area of work is further decomposed into discrete moves or techniques that can be examined, practiced, and refined.
Decomposition of leading a group discussion
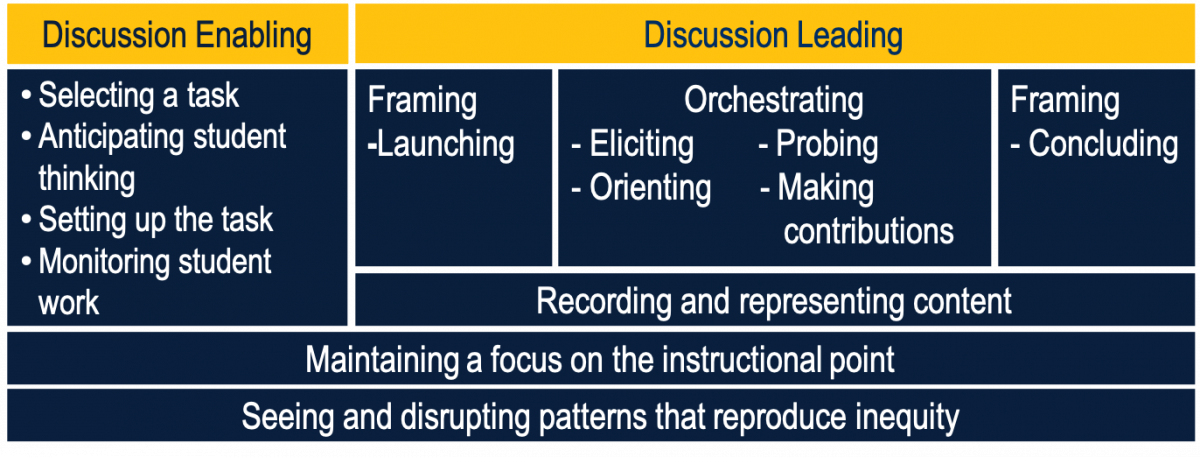
Drawing on the decomposition chart above, discussion-enablingwork includes both work the teacher does before the lesson begins, as well as the work that happens after they launch the task. Prior to the lesson, the teacher chooses a task that is not only “discussable,” but also content that is worthy of discussing, identifies the mathematical point, and anticipates what students might do. After they launch the task, the teacher circulates and engages with students as they complete the task in order to probe student ideas, move student thinking forward, and gather information about student thinking for use during the discussion.
Discussion-leadingwork includes both the framing the teacher does to launch and conclude the discussion, as well as the work of orchestrating the discussion. During orchestration, the teacher elicits student thinking by asking probing questions that help get student thinking out onto the table, and employs orienting moves to help students connect to and build off one another’s mathematical ideas. Throughout the course of leading the discussion, the teacher’s decisions are driven by a commitment to maintaining an emphasis on the mathematical point. Finally, teachers record and represent the content of the discussion publicly, in order to support all students in having access to the ideas that are being shared.
Note that the work of leading a group discussion occurs in pursuit of the goal of building collective knowledge about mathematics.
What is challenging about learning this practice?
Many of us come to our roles as educators and teacher educators with strongly held beliefs about what it means to teach and learn mathematics based on our own experiences as learners of math. When we understand doing math as primarily about getting correct answers and teaching math as conveying procedures for arriving at correct answers, we may have strong inclinations to evaluate and remediate student thinking. As a result we may miss opportunities to identify and build upon students’ existing knowledge and understanding, neglect innovative and mathematically rich student strategies, and fail to recognize the logic underlying students’ misconceptions or errors. Ultimately, these inclinations can lead us to over-utilize certain teaching approaches that reduce the cognitive work students do, such as direct instruction. By utilizing a group discussion as a means to build collective knowledge, novices can develop first the skills and eventually the dispositions to probe student ideas, orient students to one another’s ideas, make strategic contributions that maintain a focus on the mathematical point, and maintain accurate public records. Moreover, the practice is central to teaching math in a way that honors all students’ contributions and positions all students as sense-makers capable of doing meaningful mathematical work.